Guess the Chords: How to Harmonize a Melody | Hub Guitar
Hub Guitar

Guess the Chords: How to Harmonize a Melody

Harmonizing a melody with a set of chords is a skill that takes time to develop. The results are dependent on the melody, which for the purpose of harmonization could be either friendly or difficult to work with.

In this lesson, we’ll take a look at some of the more important considerations for harmonizing a melody. We’ll look at different examples, and we’ll play them on guitar.

In a Nutshell

We will take a “bare” melody, and figure out how to add chords to it.

Before Beginning

We want a rough answer to a few questions:

Operating Procedures for Choosing Chords

  • It is desirable to have enough consonance so that the result will sound like music and not garbage.

  • It is desirable to have enough dissonance so that the result will sound like music and not a string of mechanical arpeggios.

  • It is likely that several notes will be played for each chord. Even if a single measure has 5 melody notes, it quite likely it only has one chord.

  • For each chord, the note relationship could be:
    1. Melody note in the chord (most consonant)
    2. Melody note is a diatonic tension of the chord (somewhat consonant)
    3. Melody note is in the key, but not chord tone or tension (dissonant)
    4. Melody note is none of the above (most dissonant)

  • The dissonance of a note is modified by the prominence of the note, which is affected by the following:
    1. The metric position of the note’s attack and whether it falls on a strong beat, weak beat, or in between. Notes falling on a strong beat have more prominence than notes falling on a weak beat, which have more prominence than notes between beats. This relates to harmonic stress.
    2. The length of the note. Longer notes are more prominent than short notes.
    3. The amplitude of the note. Accented notes have more prominence than soft ones.

  • Leaps in the melody tend to be better supported if both notes in the leap are also in the chord.

  • Dissonances in the melody tend to be better supported if they resolve to a note that is in the chord.

Coda

To harmonize a melody, we decide how often the chord will change, roughly. If the chord will change once per measure, then every note in the measure is considered for the purpose of choosing the chord. We want to choose a chord that supports the melody, but the melody may have notes that are not in the chord and sound dissonant.

Highly dissonant notes can be managed more easily if they are in a weak metric position, they are short, or they are low in volume.

Examples of Harmonized Melodies

Example 1, No Chords

Here we have a very typical melody that follows many of the conventions established hundreds of years ago by classical music composers. The melody was clearly written with the rules of harmony in mind.

First, it looks like this melody is in C major, so we will use the chords from C major to guide our harmonization. And since the melody is simple, we’ll probably only need one chord per measure.

ImajIIminIIIminIVmajVmajVIminVIIdim

The best fit for measure 1 is probably Cmaj, because 3 out of 4 notes (C, E, G) are in the Cmaj chord. The only note not in the chord is approached and left stepwise.

Measure 2 is likely Dmin, for similar reasons. Both notes in the leap are in Dmin as well.

Measure 3 is likely Amin. Only the A and C notes are in the chord, but they fall on the metrically strong beats 1&3, whereas the other notes fall on metrically weaker beats 2&4. In addition, the melody moves by step.

Measure 4 needs two chords. The first two notes are a cadenceA resolution, or feeling of finality, created by moving from a strongly tense and unstable chord, to a stable one. Usually supported by the notes in the melody as well. The most common cadence is V–I., calling for a Gmaj chord. The last note calls for the tonic Cmaj again.

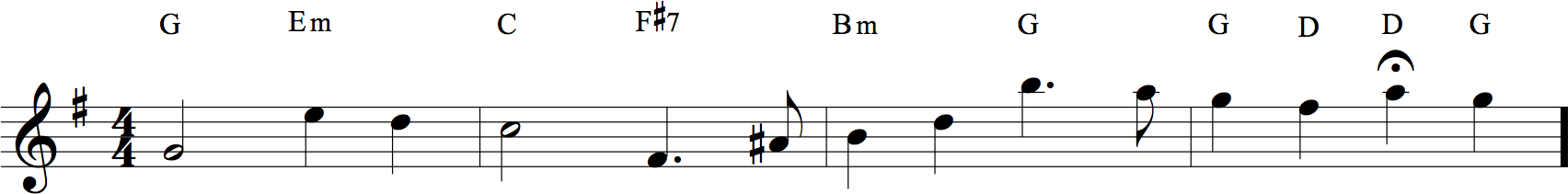
Example 2, No Chords

This G major melody is slightly more complex, and also includes an A♯ which is outside of the key. Especially for that note, we’ll want to think of chords outside of the key that might support that note. Otherwise it sounds a little random.

To increase our options, we’ll use two chords per measure.

The first measure can be Gmaj and Emin. This helps support the leap, which would otherwise leap from a chord tone of G to some weird note.

The second measure could be Cmaj and F♯7. This way the “weird” note A♯ is supported. It also happens to be the V/III, and III is the next chord.

The third measure can be Bmin and G.

Finally, to make sure the cadence goes smoothly, we can change the chord on every note if we want.

Try It At Home

Here are two examples to try yourself. Be sure to find a way to hear the results of your choices, either by playing them on guitar somehow, or maybe even playing them on piano or entering them into computer software.

Example 3, No Melody

Example 4, No Melody

Not a science

There may be many correct answers. The answers given here arose out of some of the more obvious possibilities which would come from trying to follow the process outlined above. But the goal is just to find some chords that sound good and fit nicely. It’s as simple as that.

Note

Sometimes you will find that the melody does not really fit well with any chords that you can think of. You may not have considered all of the possible chords. There are many, especially if you include secondary dominant chords and modal interchange chords. Another possibility is that maybe the melody isn’t a very good one, at least from the standpoint of harmonizing with chords. Is it possible to change the melody? Or write a bassline instead of a chord progression?

Key Tasks

  • Write your own chords to the above two melodies (4 and 5).
As the creator of Hub Guitar, Grey has compiled hundreds of guitar lessons, written several books, and filmed hundreds of video lessons. He teaches private lessons in his Boston studio, as well as via video chat through TakeLessons.

©2018 Hub Guitar. All rights reserved.